Ten Percent Of One Million

The concept of percentages is fundamental in mathematics and is widely used in various aspects of life, including finance, statistics, and everyday calculations. To understand what ten percent of one million is, we first need to break down the components of the question. One million is equal to 1,000,000. A percentage represents a part of a whole as a fraction of 100. Therefore, ten percent means 10 out of 100, or 10/100, which simplifies to 0.10 when converted to a decimal.
Calculating Ten Percent of One Million

To find ten percent of one million, we multiply one million by 0.10 (the decimal form of ten percent). This calculation is straightforward: 1,000,000 * 0.10 = 100,000. Thus, ten percent of one million is 100,000.
Practical Applications and Examples
Understanding percentages and being able to calculate them is crucial in numerous real-world scenarios. For instance, if you were to invest one million dollars in a venture that yields a ten percent return, you would expect to earn 100,000 from that investment. Similarly, in sales, if a product originally priced at one million dollars is discounted by ten percent, the discount amount would be 100,000, making the sale price $900,000.
| Calculation Component | Value |
|---|---|
| Original Amount | 1,000,000 |
| Percentage Rate | 10% |
| Decimal Form of Percentage | 0.10 |
| Calculated Amount (10% of 1,000,000) | 100,000 |

Key Points
- To find ten percent of a number, multiply that number by 0.10.
- Ten percent of one million equals 100,000.
- Percentages are used in finance, statistics, and everyday calculations to represent parts of a whole.
- Understanding how to calculate percentages is crucial for making informed decisions in investments, sales, and other areas.
- The decimal form of a percentage is obtained by dividing the percentage value by 100.
Implications and Further Considerations
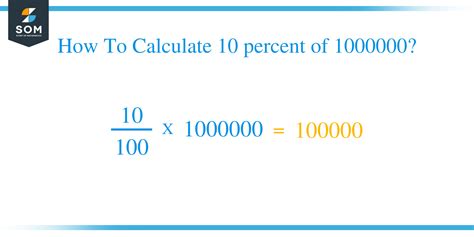
The ability to calculate percentages is not only a mathematical skill but also a practical tool for navigating financial and statistical information. Whether it’s calculating interest rates, understanding discounts, or analyzing growth rates, percentages provide a common language for discussing proportions and changes. The calculation of ten percent of one million, resulting in 100,000, is a basic yet foundational example of how percentages work and can be applied in real-world contexts.
As we delve deeper into the world of percentages and financial calculations, it becomes clear that understanding these concepts is vital for making informed decisions. Whether you are an investor looking to understand returns on investment, a consumer trying to decipher sales and discounts, or a student seeking to grasp mathematical concepts, knowing how to work with percentages is an indispensable skill.
Evolving Trends and Technologies
In an era where data analysis and financial literacy are increasingly important, the role of percentages in facilitating understanding and decision-making cannot be overstated. With the advent of digital tools and calculators, performing percentage calculations has become easier than ever. However, the fundamental principles behind these calculations remain unchanged, emphasizing the importance of a solid grasp of mathematical concepts for navigating an increasingly complex world.
What is the formula to calculate a percentage of a number?
+The formula to calculate a percentage of a number is: (percentage / 100) * number. For example, to find ten percent of one million, you would calculate (10 / 100) * 1,000,000 = 0.10 * 1,000,000 = 100,000.
How are percentages used in real-life scenarios?
+Percentages are used in a variety of real-life scenarios including finance (interest rates, investment returns), sales (discounts), statistics (growth rates, proportions), and more. They provide a way to express a part of a whole as a fraction of 100, making it easier to understand and compare different values.
Why is understanding percentages important?
+Understanding percentages is important because it allows individuals to make informed decisions in various aspects of life, from personal finance to professional endeavors. It facilitates the comparison of proportions, the calculation of changes, and the analysis of data, thereby empowering individuals with the knowledge needed to navigate complex financial and statistical information.
In conclusion, the calculation of ten percent of one million, yielding 100,000, serves as a foundational example of how percentages work and their practical applications. As we continue to navigate an increasingly complex world filled with financial and statistical data, the importance of understanding percentages and how to calculate them will only continue to grow. By grasping these mathematical concepts and applying them in real-world scenarios, individuals can make more informed decisions, contributing to personal and professional success.



